Antenna
Design
Lab
07 - Friis Transmission Equation
Dipole dimensions:
Feeding: Center-fed with a discrete
port with source impedance of 50 W
Design frequency: f 0 =
(1000 + PIN/1000) MHz=1GHz
실습조교 PIN =
0000
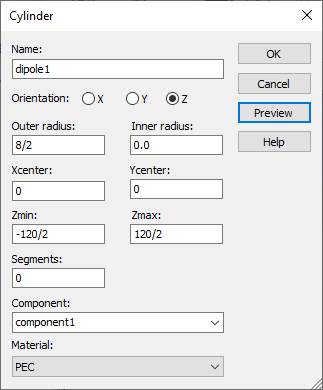
Dipole end-to-end length: L =
0.40 λ=120mm
Dipole diameter: d = L/15=8mm
Dipole feed gap at the dipole center: g = d/2=4
Dipole material: PEC
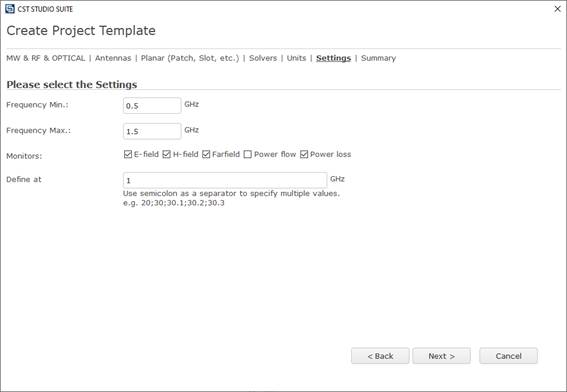
Frequency range: 0.5f0 to 1.5f0
Use Dipole #1 and Dipole #2 with dimensions as above.
Dipole #1 arm: Direction = z, dipole center
at y = 0 and x = 0
Dipole #2 arm: Direction = z, dipole center
at y = R and x = 0
ㅇ 프로젝트 template 생성
ㅇ 다이폴 #1 구조 그리기: 다이폴 원통 전체를 만든 후, 급전 간극 (feed gap)을 제거한다.
(1) Make a Dipole 1
Modeling, Cylinder 아이콘 선택, ESC 키, Name:
solid1, Orientation: Z
(2) Make a feed gap
Modeling, Cylinder 아이콘 선택, ESC 키, Name:
solid1, Orientation: Z

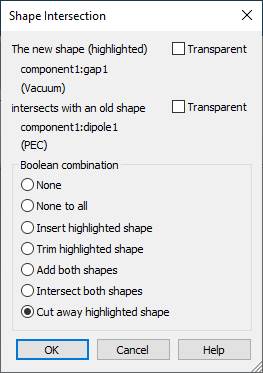
Next
Shape intersection: Cut away
highlighted shape
(3) Make a Dipole 2
ㅇ 다이폴 #2 구조 그리기: 다이폴 #1을 복사한다.
Use Dipole #1 and Dipole #2 with dimensions as above.
Dipole #1 arm: Direction = y, dipole center
at z = 0 and x = 0
Dipole #2 arm: Direction = y, dipole center
at z = R and x = 0
a) Select the dipole1

b) Make dipole 2
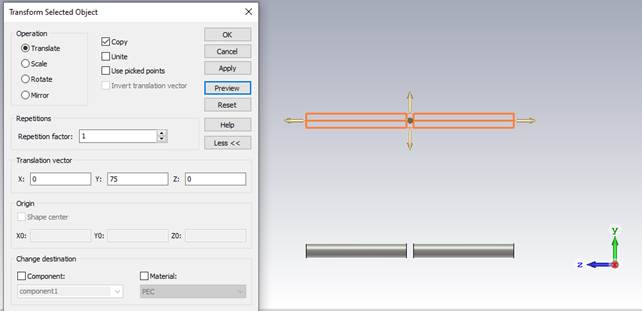
wire using Transform
For R = 0.25 λ=0.25*300=75 mm
Modelling, Components, component1
Click the dipole 1, the right mouse button, Transform
X: 0
Y:75
Z: 0

ㅇ 다이폴 급전 포트 설정
Modeling, Pick Points, Pick Face
Center, gap 한면에 마우스 위치후 더블클릭
Pick Points, Pick Face Center, gap 한면에 마우스 위치후 더블클릭
Simulation, Discrete Port
키보드 A를 누르고 중앙 선택
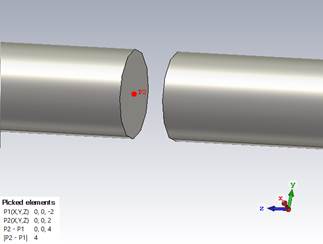
- 아래와 같이 점이 생성됨
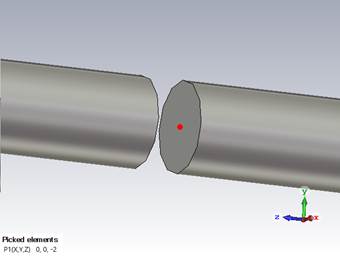
- 위와 같은 방식으로 반대쪽에도 점 생성
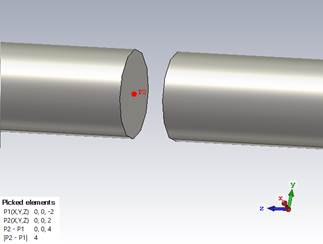
-
[Simulation]-[Discrete Port] Click
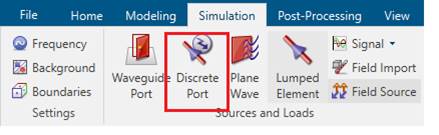
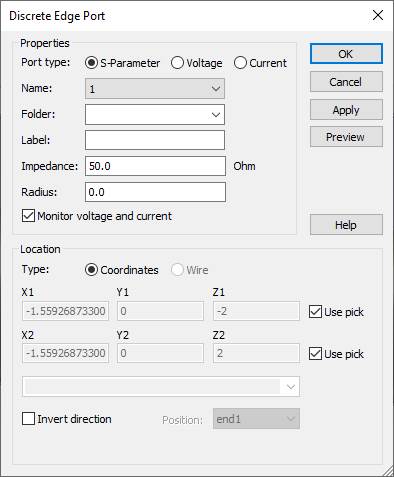
- 아래와 같은 창이 생성됨
- OK
Click
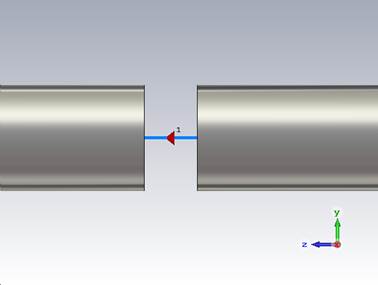
- 반대쪽도 똑같이 생성해주면 된다.
- 유의할 점은 포트의 방향은 같아야 한다는 것.
Repeat a discrete port2
same the discrete port1.
ㅇ 시뮬레이션 설정
주파수 설정:
Simulation, Frequency, Min. frequency:
0.5, Max. frequency: 1.5
필드 모니터 설정:
Simulation, Field Monitor, E-field,
Frequency, Frequency:1, Apply
Simulation, Field Monitor, H-field and
Surface current, Frequency, Frequency:1, Apply
Simulation, Field Monitor, Far
field/RCS, Frequency, Frequency:1, Apply
Simulate
Simulation, Setup Solver, Start
1-1. Plot the antenna structure.
1-2. Plot Plot |S11|, |S21|
in dB.
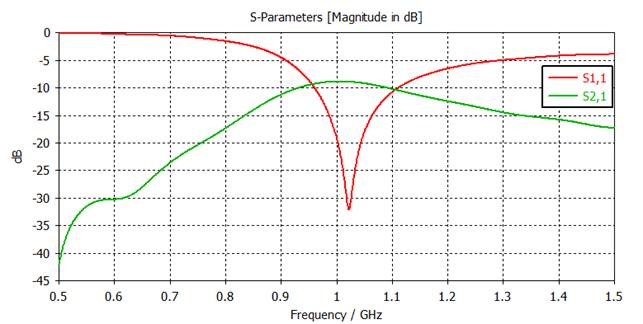
1-3. Find |S21| (dB) at f0.
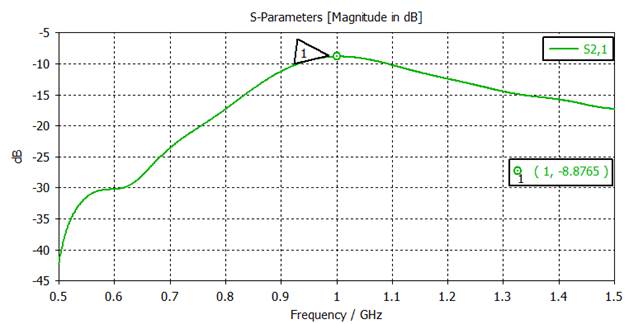
|S21| =
-8.87 dB @ 1GHz
1-4. Plot the co-polarized gain Gtheta
at f0 of a dipole toward the other dipole on a
polar plot in θ= 90° plane.
Find the Gtheta (dB) in the direction of the other dipole. The presence of the
other dipole may change the omnidirection pattern of an isolated dipole.
- Navigation
Tree,
2D/3D Results, Farfileds, Theta
a) Polar
- 3D
pattern에서 마우스 우클릭 후 [Farfield
Plot Properties] 클릭
- 아래 화면과 같이 지정
(Theta->Phi,
OK)
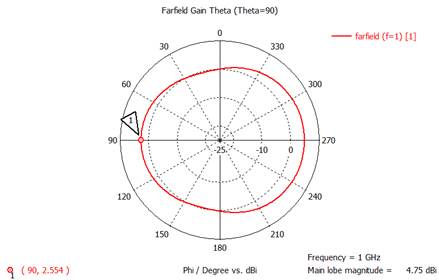
Gtheta = 2.55 dBi @ phi = 90° (in the
direction of the other antenna)
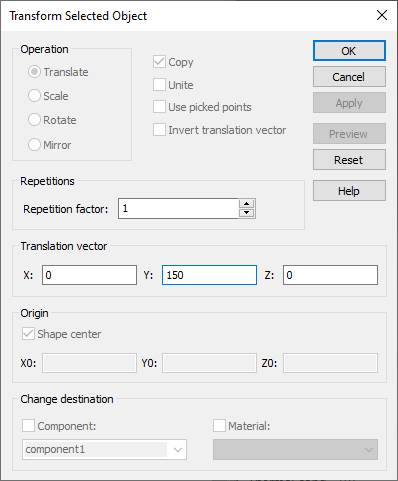
For Y=R = 0.5 λ=150
mm, repeat the above.
Double click the dipole1_1

Then double click the Transform
component (translate)

Change the distance (Y=R =
0.5 λ=150 mm)
Simulate
Simulation, Setup Solver, Start
2-1.Plot
the antenna structure.
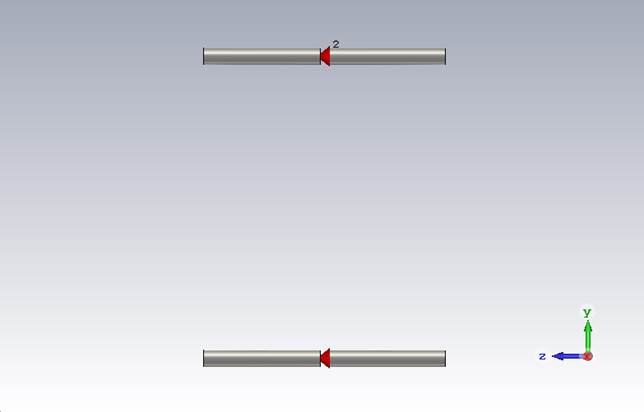
2-2. Plot Plot |S11|, |S21|
in dB.
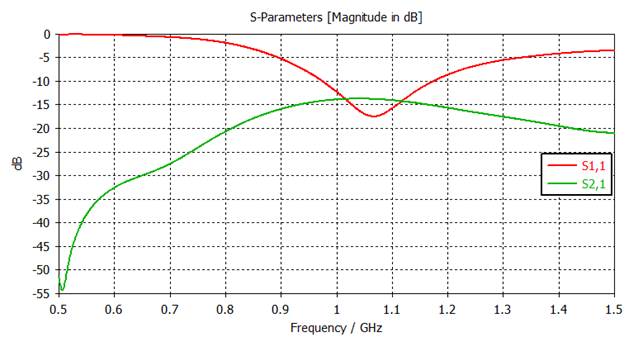
2-3. Find |S21| (dB) at f0.
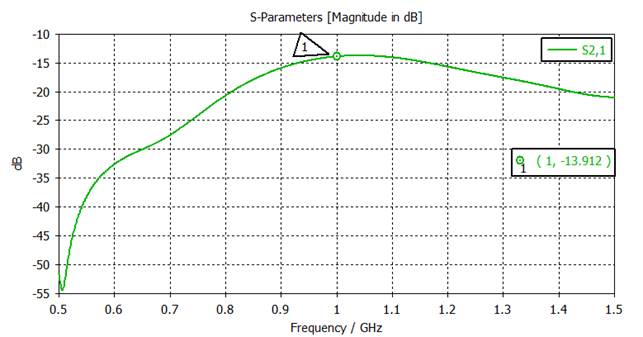
|S21| =
-13.91 dB @ 1GHz
2-4. Plot the co-polarized gain Gtheta at f0 of
a dipole toward the other dipole on a polar plot in θ= 90° plane. Find the Gtheta (dB) in the direction of the
other dipole. The presence of the other dipole may change the omnidirection
pattern of an isolated dipole.
a) Polar
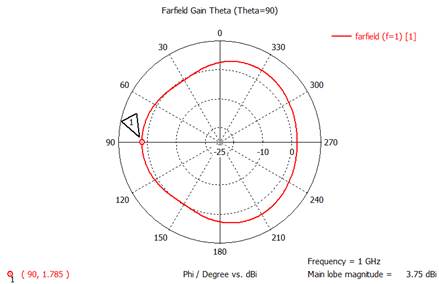
Gtheta = 1.79 dBi @ 1 GHz in the direction of the other antenna
For Y= R = 1.0 λ=300
mm, repeat the above.
Double click the dipole1_1

Then double click the Transform
component (translate)

Change the distance (Y = R =
1.0 λ=300 mm)
Simulate
Simulation, Setup Solver, Start
3-1.Plot
the antenna structure.
3-2. Plot Plot |S11|, |S21|
in dB.
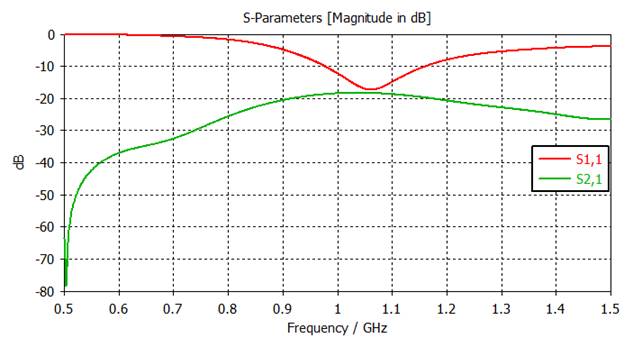
3-3. Find |S21| (dB) at f0.
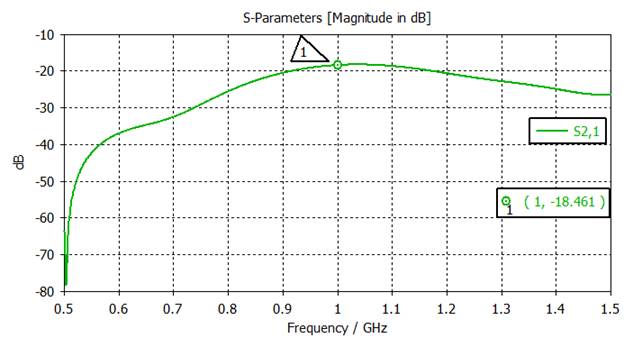
|S21| =
-18.46 dB @ 1GHz
3-4. Plot
the co-polarized gain Gtheta at f0 of a dipole
toward the other dipole on a polar plot in θ=
90° plane. Find the Gtheta (dB) in the direction of the other dipole. The presence
of the other dipole may change the omnidirection pattern of an isolated dipole.
a)
Polar
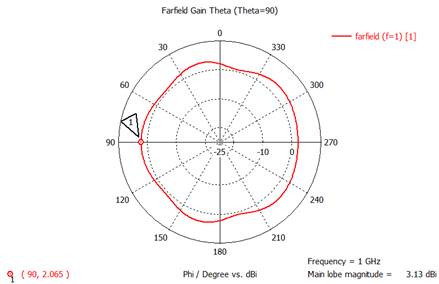
Gtheta = 2.07 dBi @ 1 GHz in the direction of the other antenna
II.
Discussions
1.
Compare |S21| (dB) by CST Studio with |S21| (dB) by Friis equation.
|
R = 0.25 λ |
R = 0.5 λ |
R = 1.0 λ |
|
|
1. |S21| (dB), CST Studio |
-8.87 |
-13.91 |
-18.46 |
|
2. Antenna gain Gtheta (dB) in the other dipole
direction |
2.55 |
1.79 |
2.07 |
|
3. Path loss: (λ/4πR)2
(dB) |
1/(4π×0.25)2 = -9.94 |
1/(4π×0.5)2 = -15.94 |
1/(4π×1.0)2
= -21.94 |
|
4. |S21| (dB), Friis equation |
-4.84 |
-12.36 |
-17.80 |
|
5. |S21| difference (dB) (1-4) |
-4.03 |
-1.55 |
-0.66 |
2.
Find the minimum distance where the Friis equation is accurate within ±1
dB. Note the Friis equation is accurate when both antennas lie in the far-field
region of the other antenna.
R = 0.5, delta =
-1.55
R = 1.0, delta =
-0.66
R = x, delta = -1.0
-------------------------------
Use a linear approximation (비례식)
x = 1.0 - (1.0-0.66)*(1.0-0.5)/(1.55-0.66) = 0.809 λ